
Contributed by Dr. Gui Zhou and Dr. Cunhua Pan based on IEEEXplore® article, A Framework of Robust Transmission Design for IRS-aided MISO Communications with Imperfect Cascaded Channels and an SPS webinar available in the SPS Resource Center.

Fig. 1. Schematic diagram of passive RIS circuit.
Introduction
Reconfigurable intelligent surface (RIS), which is also known as intelligent reflecting surface (IRS) or large intelligent surface (LIS), has emerged as a promising technique to enhance the spectral and energy efficiency of the wireless networks, thanks to its artificial planar passive radio array structure which is cost-effective and energy-efficient. As shown in Fig. 1, each element on the RIS is capable of reconfiguring the wireless environment between the base station (BS) and users constructively or destructively by imposing an independent phase shift to the incident signal. The existing literature on RIS-aided wireless communications has demonstrated that RIS is an enabler for enhancing the spectral and energy efficiency through jointly optimizing the beamforming at the BS and the phase shifts at the RIS. However, most existing contributions on transceiver design are based on the ideal assumption of accurate RIS-related channel state information (CSI), which is challenging in practice. Specifically, let us first recall the channel estimation procedure for the RIS-aided communication systems, which consists of two phases as shown in Fig. 2. At Phase I, the BS estimates the direct CSI between the BS and users by switching off the RIS. At Phase II, the BS estimates the overall CSI (the summation of the direct CSI and RIS-related CSI) and then acquires the RIS-related CSI by subtracting direct CSI from the overall CSI. Since the direct CSI has inevitable channel estimation error, the acquired RIS-related CSI suffers from the error propagation, which would cause severe quality of service (QoS) outage. Hence, it is imperative and crucial to consider the robust transmission design by considering the channel estimation error of the RIS-related CSI.
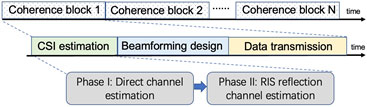
Fig. 2. Frame structure.
Although robust transmission design in wireless communications has been extensively studied in the past two decades, it has not been well investigated for RIS-aided communication system. One may consider the robust design under the imperfect individual RIS-related channels (BS-RIS channels or/and RIS-user channels) as studied in [2]. However, since the RIS is equipped with passive reflecting elements without radio frequency (RF) chains, the RIS is not capable of estimating these individual channels. Fortunately, it is found that the cascaded BS-RIS-user channels, which are the product of the BS-RIS channel and the RIS-user channels, are sufficient for the transceiver design. Hence, most of the existing contributions focused on the cascaded channel estimation, which results in sizeable cascaded channel error due to error propagation and limited pilot transmission power. In order to solve this practical problem, our work in [1] proposed a framework of robust beamforming design for RIS-aided systems with imperfect cascaded channels.
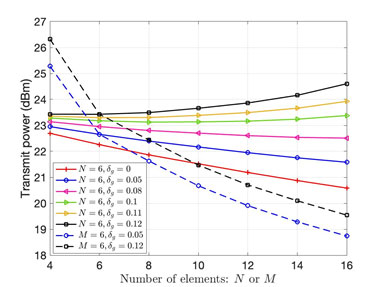
Fig. 3. Transmit power versus the number of antennas at the BS (N) or elements at the RIS (M) [1].
1. Outage constrained robust beamforming design under statistical CSI error model
In general, the conventional minimum mean square error (MMSE) method is generally adopted to estimate the cascaded channel, and thus the estimated channel and its estimation error generally follow the circularly symmetric complex Gaussian (CSCG) distribution. For example, in the time division duplex (TDD) operation mode, noise and limited pilot training power will cause inevitable channel estimation error, which generally follows the complex Gaussian distribution. Hence, we adopt the statistical CSI error model to characterize the channel estimation error. Specifically, we aim to minimize the total transmit power of the BS under the rate outage constraints where the probability of each user that can successfully decode its message at a target data rate is no less than a predefined maximum data rate outage probability. The outage constrained robust beamforming design problem is computationally intractable due to the fact that the rate outage probability constraints have no simple closed-form expressions. Thus, we adopt a safe approximation based on Bernstein-type inequality to tackle the rate outage constraints and semidefinite relaxation (SDR) method to solve the resulting convex semidefinite program (SDP) problem with rank-one property. It is observed from Fig. 3 that the level of the cascaded BS-RIS-user (BRU) channel error plays a vital role in the RIS-aided systems. Specifically, when the cascaded BRU error is small, the total transmit power decreases with the number of the reflecting elements due to the increased beamforming gain. However, when the cascaded BRU error is large, the transmit power increases with the number of the reflecting elements due to the increased level of channel estimation error. This conclusion provides a useful design insight: whether to deploy the RIS depends on the level of the cascaded BRU error. If the channel estimation error is large, it is not beneficial to deploy the RIS in the wireless networks.

Fig. 4. Outage probability of rate versus the channel uncertainty level δ. The y-axis stands for the probability that the target rate of at least one user is not satisfied [2].
2. Worst-case robust beamforming design under bounded CSI error model
When the channel error is caused by the quantization error, its error power naturally belongs to a bounded region. For example, in the frequency division duplex (FDD) operation mode, the receiver estimates the downlink channel and then feeds the rate-limited quantized CSI back to the transmitter. Then, the acquired CSI is plagued by quantization errors. The worst-case robust beamforming design is considered under the bounded CSI error model. We aim to minimize the total transmit power of the BS by the joint design of the precoder matrix and reflection beamforming vector under the unit-modulus constraints and the worst-case QoS constraints, i.e., ensuring the achievable rate of each user to be above a threshold for all possible channel error realizations. In order to solve the non-convex robust design problem with semi-infinite inequality constraints and coupled variables, an algorithm is proposed based on S-Procedure, second-order cone programming (SOCP) and penalty convex-concave procedure (CCP). As can be observed in Fig. 4, when the beamforming design ignores the channel error, the target rate of at least one user is generally not met, especially for high channel uncertainty level and high target rate. However, our adopted worst-case robust beamforming design method can ensure that no outage occurs.
This work provides a framework of robust transmission design in a simple single-cell multiuser scenario. Based on this framework, we have addressed the robust transmission design for the RIS-assisted secure communication systems [3] and RIS-aided cognitive radio systems [4].
References:
[1] G Zhou, C. Pan, H. Ren, K. Wang and A. Nallanathan, “A Framework of Robust Transmission Design for IRS-Aided MISO Communications With Imperfect Cascaded Channels,” IEEE Trans. Signal Processing, vol. 68, pp. 5092-5106, Aug. 2020. doi: https://dx.doi.org/10.1109/TSP.2020.3019666.
[2] G Zhou, C. Pan, H. Ren, K. Wang, M. Di Renzo and A. Nallanathan, “Robust Beamforming Design for Intelligent Reflecting Surface Aided MISO Communication Systems,” IEEE Wireless Commun. Lett., vol. 9, no. 10, pp. 1658-1662, Oct. 2020. doi: https://dx.doi.org/10.1109/LWC.2020.3000490.
[3] S. Hong, C. Pan, H. Ren, K. Wang, K. K. Chai and A. Nallanathan, “Robust Transmission Design for Intelligent Reflecting Surface-Aided Secure Communication Systems With Imperfect Cascaded CSI,” IEEE Trans. Wireless Commun., vol. 20, no. 4, pp. 2487-2501, Apr. 2021, doi: https://dx.doi.org/10.1109/TWC.2020.3042828.
[4] L. Zhang, C. Pan, Y. Wang, H. Ren and K. Wang, “Robust Beamforming Design for Intelligent Reflecting Surface Aided Cognitive Radio Systems With Imperfect Cascaded CSI,” IEEE Trans. Cognitive Commun. Networking, vol. 8, no. 1, pp. 186-201, Mar. 2022, doi: https://dx.doi.org/10.1109/TCCN.2021.3107510.
